SCIENTIFIC COMPUTING AND IMAGING INSTITUTE
at the University of Utah

SCI Publications

2003

A.A. Samsonov, E.G. Kholmovski, C.R. Johnson. “Determination of the Sampling Density Compensation Function Using a Point Spread Function Modeling Approach and Gridding Approximation,” In Proceedings of 11th Annual Meeting of the International Society for Magnetic Resonance in Medicine (ISMRM), Toronto, pp. 477. 2003.

A.A. Samsonov, E.G. Kholmovski, C.R. Johnson. “A Modified POCSENSE Technique for Accelerated Iterative Reconstruction from Sensitivity Encoded MRI Data,” In Proceedings of 11th Annual Meeting of the International Society for Magnetic Resonance in Medicine (ISMRM), Toronto, pp. 477. 2003.

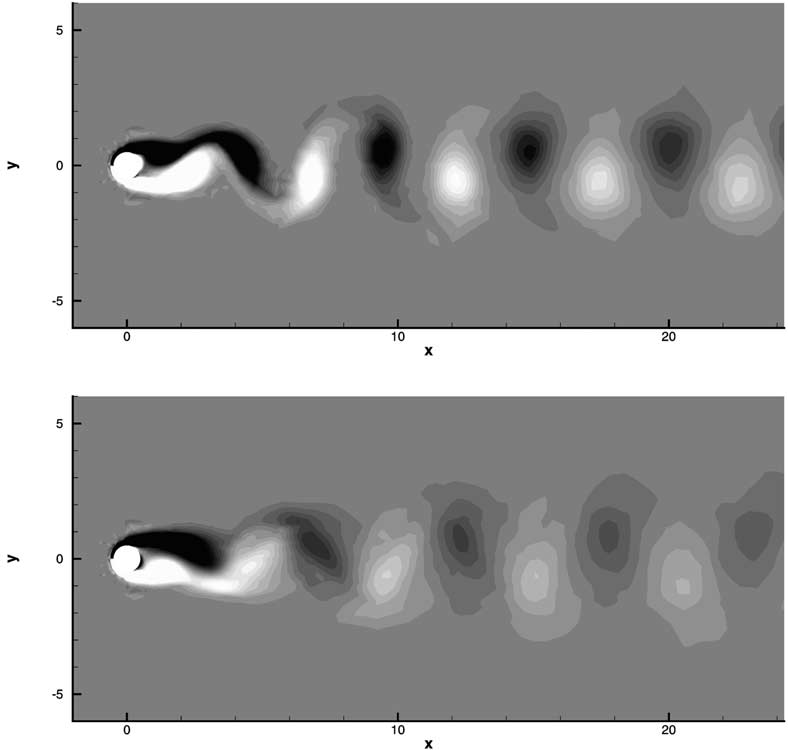
A.R. Sanderson, C.R. Johnson. “Display of Vector Fields Using a Reaction-Diffusion Model,” SCI Institute Technical Report, No. UUSCI-2003-002, University of Utah, June, 2003.

J. Schpok, J. Simons, D.S. Ebert, C.D. Hansen. “A Real-Time Cloud Modeling, Rendering, and Animation System,” In ACM Symposium on Computer Animation, San Diego, pp. 160--166. 2003.

Y. Serinagaoglu, D.H. Brooks, R.S. MacLeod. “Prior Model Selection for Bayesian Inverse Electrocardiography,” In Proceedings of the NFSI Conference 2003, Chieti, Italy, 2003.

Y. Serinagaoglu, R.S. MacLeod, D.H. Brooks. “A Bayesian Approach to Inclusion and Performance Analysis of Using Extra Information in Bioelectric Inverse Problems,” In Proceedings of the IEEE International Conference on Image Processing, 2003.

Y. Serinagaoglu, D.H. Brooks, R.S. MacLeod. “Combining Numerical and Physiological Constraints in Inverse Electrocardiography,” In Int. J. Bioelectromag., Vol. 5, No. 1, pp. 215--217. 2003.

J.G. Stinstra, B. Hopenfeld, R.S. MacLeod. “A Model for the Passive Cardiac Conductivity,” In Int. J. Bioelectromag., Vol. 5, No. 1, pp. 185--186. 2003.

M. Styner, G. Gerig, S. Pizer, S. Joshi. “Automatic and Robust Computation of 3D Medial Models Incorporating Object Variability,” In International Journal of Computer Vision - Special UNC-MIDAG issue, Vol. 55, No. 2, Edited by O Faugeras and K Ikeuchi and J Ponce, Kluwer Academic, pp. 107--122. November-December, 2003.

T. Tasdizen, R.T. Whitaker, P. Burchard, S. Osher. “Geometric Surface Processing via Normal Maps,” In ACM Transactions on Graphics, 2003.

T. Tasdizen, R.T. Whitaker. “Cramer-Rao Bounds for Nonparametric Surface Reconstruction from Range Data,” In Proceedings of Fourth International Conference on 3-D Imaging and Modeling, pp. 70--77. October, 2003.

T. Tasdizen, R.T. Whitaker. “Anisotropic diffusion of surface normals for feature preserving surface reconstruction,” In Proceedings of Fourth International Conferenceon 3-D Imaging and Modeling, pp. 353--360. October, 2003.

T. Tasdizen, R.T. Whitaker. “Feature preserving variational smoothing of terrain data,” In IEEE Workshop on Variational, Geometric and Level Set Methods in Computer Vision, October, 2003.

X. Tricoche, G. Scheuermann. “Topology Simplification of Symmetric, Second Order 2D Tensor Fields,” In Geometric Modeling Methods in Scientific Visualization, Springer, Berlin, pp. 171--184. 2003.

R. Van Uitert, D. Weinstein, C.R. Johnson. “Volume Currents in Forward and Inverse Magnetoencephalographic Simulations Using Realistic Head Models,” In Annals of Biomedical Engineering, Vol. 31, pp. 21--31. 2003.

R. Van Uitert, C.R. Johnson. “Influence of Brain Conductivity on Magnetoencephalographic Simulations in Realistic Head Models,” In The 25th Annual International Conference of the IEEE Engineering In Medicine And Biology Society, Vol. 3, pp. 2136--2139. September, 2003.

D.M. Weinstein, J.V. Tranquillo, C.S. Henriquez, C.R. Johnson. “BioPSE Case Study: Modeling, Simulation, and Visualization of Three Dimensional Mouse Heart Propagation,” In International Journal of Bioelectromagnetism, Vol. 5, No. 1, pp. 314--315. 2003.

C. Wyman, C.D. Hansen. “Penumbra Maps: Approximate Soft Shadows in Real-Time,” In Eurographics Rendering Symposium, Belguim, pp. 202--207. 2003.

D. Xiu, G.E. Karniadakis. “Modeling Uncertainty in Flow Simulations via Generalized Polynomial Chaos,” In Journal of Computational Physics, Vol. 187, No. 1, pp. 137--167. 2003.
DOI: 10.1016/S0021-9991(03)00092-5

×

We present a new algorithm to model the input uncertainty and its propagation in incompressible flow simulations. The stochastic input is represented spectrally by employing orthogonal polynomial functionals from the Askey scheme as trial basis to represent the random space. A standard Galerkin projection is applied in the random dimension to obtain the equations in the weak form. The resulting system of deterministic equations is then solved with standard methods to obtain the solution for each random mode. This approach can be considered as a generalization of the original polynomial chaos expansion, first introduced by Wiener [Am. J. Math. 60 (1938) 897]. The original method employs the Hermite polynomials (one of the 13 members of the Askey scheme) as the basis in random space. The algorithm is applied to micro-channel flows with random wall boundary conditions, and to external flows with random freestream. Efficiency and convergence are studied by comparing with exact solutions as well as numerical solutions obtained by Monte Carlo simulations. It is shown that the generalized polynomial chaos method promises a substantial speed-up compared with the Monte Carlo method. The utilization of different type orthogonal polynomials from the Askey scheme also provides a more efficient way to represent general non-Gaussian processes compared with the original Wiener–Hermite expansions.

Keywords: Polynomial chaos, Uncertainty, Fluids, Stochastic modeling

D. Xiu, G.E. Karniadakis. “A New Stochastic Approach to Transient Heat Conduction Modeling with Uncertainty,” In International Journal of Heat and Mass Transfer, Vol. 46, No. 24, pp. 4681--4693. 2003.
DOI: 10.1016/S0017-9310(03)00299-0